Introduction of square
A square may be a two-dimensional figure with four equal sides and every one of the four angles square measures an adequate ninety degrees. The properties of a parallelogram square measure somewhat the same as an sq., however the distinction between the 2 is, that a parallelogram has solely its opposite sides equal. Therefore, a parallelogram is termed an sq. as long as all its four sides square measure of equal length.
Square Number of sides = four Number of vertices = four Area = Side2 Perimeter = 4(Side) The other properties of the SQL. like space and perimeter additionally disagree from that of a parallelogram. allow us to learn here well, what's an sq. and its properties at the side of solved examples.
Definition
A Square may be a regular quadrilateral that has all four sides of equal length and every one four angles are equal. The angles of the square measure at right-angle or adequate 90-degrees. Also, the diagonals of the sq. square measure equal and cut one another at ninety degrees.
An sq. can even be outlined as a parallelogram wherever 2 opposite sides have equal length.
The on top of the figure represents an sq. wherever all the perimeters square measure equal and every angle equals ninety degrees.
Just like a parallelogram, we will additionally take into account a parallelogram (which is additionally a protrusive quadrilateral and has all four sides equal), as an sq., if it's a right vertex angle. In the same means, a quadrangle with all its 2 adjacent equal sides and one right vertex angle maybe an sq...
1. Shape Of sq.
An sq. may be a quadrilateral polygonal shape that has its all sides equal long and also the lives of the angles square measure ninety degrees. The form of the sq. is like, if it's cut by a plane from the middle, then each half-square measure is symmetrical. every 1/2 the sq. then feels like a parallelogram with opposite sides equal.
2. Properties of sq.

properties-sq
The most necessary properties of an sq. square measure are listed below: All four interior angles square measure an adequate 90° All four sides of the sq. square measure congruent or adequate to one another The opposite sides of the sq. square measure parallel to every different The diagonals of the sq. cut one another at 90° The two diagonals of the sq. square measure adequate one another The square. has four vertices and four sides The diagonal of the sq. divide into 2 similar symmetrical triangles The length of diagonals is larger than the perimeters of the sq.
3 . Space And Perimeter Of sq.
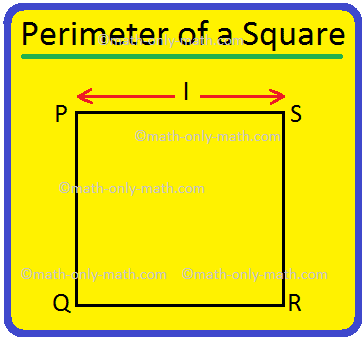
permiter-sq
The square measure and perimeter are 2 main properties that outline an sq. as an sq.. allow us to learn them one by one:
AREA
The area of the sq. is the region coated by it in an exceedingly two-dimensional plane. the realm here is adequate the sq. of facets|the edges|the perimeters} or side square. it's measured in sq. units.
space = side2 per sq. unit
Perimeter Of sq. The perimeter of the sq. is adequate to the total of all its four sides. The unit of the perimeter remains constant as that of side-length of sq...
Perimeter = facet + facet + facet + facet = four facet
Perimeter = four × facets of the sq.
If ‘a’ is the length of facet of sq., then the perimeter is:
Perimeter = 4a unit
4 . Length of Diagonal square.
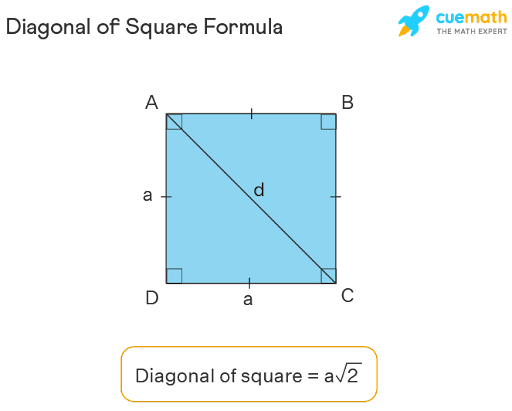
diagonal-square
The length of the diagonals of the sq. is adequate s√2, wherever s is that the facet of the sq... As we know, the length of the diagonals is adequate for one another. Therefore, by Pythagoras theorem, we can say, the diagonal is the flank, and also the 2 sides of the Triangulum fashioned by the diagonal of the square measure perpendicular and base.
Since, Hypotenuse2 = Base2 + Perpendicular2 Hence, Diagonal2 = Side2 + Side2 Diagonal = D = s√2
Where d is the length of the diagonal of a square. and s is that the facet of the sq...
5 . Diagonal square.
Diagonal of a square. maybe a line phase that connects 2 opposite vertices of the sq... As we've got four vertices of an sq., therefore we will have 2 diagonals at intervals of an sq... Diagonals of the square measure invariably bigger than its sides.
Below given square measure some necessary relation of diagonal of a square. and different terms associated with the sq...
Relation between Diagonal ‘d’ and facet ‘a’ of an sq. - d = a√2 Relation between Diagonal ‘d’ and space ‘A’ of a Square- d=√2A Relation between Diagonal ‘d’ and Perimeter ‘P’ of a Square- d=p/2√2 Relation between Diagonal ‘d’ and Circumradius ‘R’ of a square: d = 2R Relation between Diagonal ‘d’ and diameter of the Circumcircle – d=Dc Relation between Diagonal ‘d’ and In-radius ® of a circle- d=2√2r Relation between Diagonal ‘d’ and diameter of the In-circle – d=√2Di Relation between diagonal and length of the phase l – d=l 2√10/5
Description A square is a closed, two-dimensional form with four equal sides. An sq. may be a quadrilateral.
Square form
We can notice the form of an sq. in an exceedingly game board or chessboard, a clock, and in an exceeding slice of bread, around us.
Conclusion
All the squares. numbers finish with zero,1,4,6, or nine at the unit palace. sq. ranges will solely have an even number of zeros at the tip. the root is the inverse operation of sq... Their square measure 2 integral sq. roots of an ideal sq. range. The sq. operate preserves the order of positive numbers: larger numbers have larger squares. In different words, the sq. may be a monotonic open on the interval [0, +∞). On the negative numbers, numbers with bigger definite quantities have bigger squares, that the sq. may be a monotonically decreasing operation on (−∞,0].